MAKE A MEME
View Large Image
| View Original: | Rotations on the complex plane.svg (783x776) | |||
| Download: | Original | Medium | Small | Thumb |
| Courtesy of: | commons.wikimedia.org | More Like This | ||
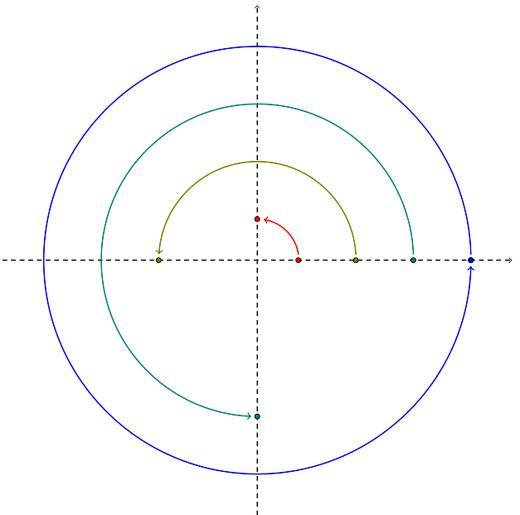
| Keywords: Rotations on the complex plane.svg Four numbers on the real line are multiplied by integer powers of the imaginary unit which corresponds to rotations by multiples of the right angle Self-made Written in TeX with TikZ compiled using <code>latex rot tex dvisvgm -n -c1 75 rot dvi</code> Ke r TeX source <source lang latex > \documentclass article \usepackage amsmath \usepackage amsfonts \usepackage tikz \usepackage scalefnt \begin document \pagestyle empty \def\rotarc 1 2 3 4 5 \draw 2 fill 2 1 0 circle 0 03 ; \draw 2 fill 2 1 cos 3 1 sin 3 circle 0 03 ; \drawthick -> 2 1 0 075 arc asin 0 075/ 1 3/2 1 node anchor 5 4 arc 3/2 3-asin 0 075/ 1 1 ; \begin tikzpicture scale 2 \drawdashed -> -3 1 0 -- 3 1 0 node anchor north Re ; \drawdashed -> 0 -3 1 -- 0 3 1 node anchor west Im ; \rotarc 0 5 red 90 i 1 i south west \rotarc 1 2 olive 180 i 2 -1 south west \rotarc 1 9 teal 270 i 3 -i south east \rotarc 2 6 blue 360 i 4 1 south east \end tikzpicture \end document </source> Mathematical diagrams Complex plane Rotation Euclidean plane Cc-zero | ||||