MAKE A MEME
View Large Image
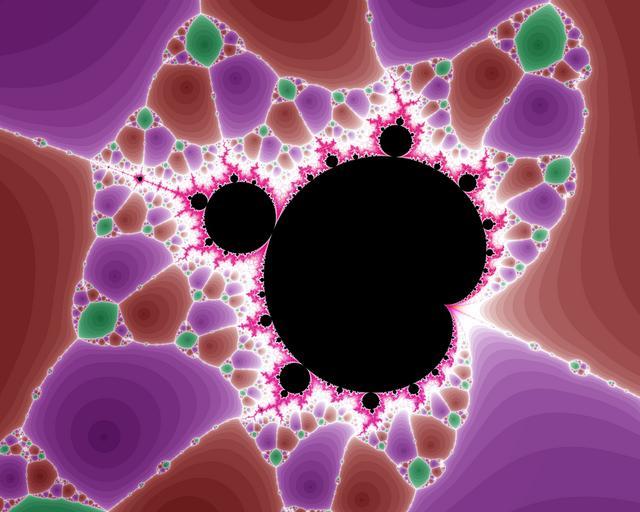
| View Original: | Newton-lplane-Mandelbrot.jpg (6000x4800) | |||
| Download: | Original | Medium | Small | Thumb |
| Courtesy of: | commons.wikimedia.org | More Like This | ||
| Keywords: Newton-lplane-Mandelbrot.jpg Computergraphical study of the critical point 0 of some polynomials <math>p_\lambda</math> under Newton's method in the complex <math>\lambda</math>-plane own 2008-04-11 Georg-Johann Lay thumb right Fate of zero one of the critical points of <math>N_ p_\lambda </math> for polynomials from the family <math> p_\lambda z\mapsto z 3+ \lambda-1 z-\lambda </math> in the complex plane <math>N</math> denotes the Newton operator <math> N_f f \mapsto \mathit id - \frac f f' </math> For <math>\lambda</math> in the black part of the plane the critical point 0 of <math>N_ p_\lambda </math> does not converge to a zero of <math>p_\lambda</math> This means that the set of start values for which Newton's method does not converge to a zero of <math>p_\lambda</math> is a set of full measure The black set is <math>M \ \lambda\in\mathbb C N_ p_\lambda k 0 \not\to \text root of p_\lambda \text as k \to\infty\ </math> The center of the region is at about <math>\lambda 0 3+1 64i</math> Mandelbrot set shapes in other fractals Newton fractals Level set method | ||||